EUCLID PRINCIPLES

Euclid's Principles, also known as Euclid's Elements, is a mathematical treatise consisting of 13 books that presents a comprehensive and systematic approach to geometry. The treatise begins with a set of definitions and postulates, which serve as the basis for all subsequent reasoning.

Euclid's postulates, also known as his axioms, form the foundation of his mathematical system in "Elements". There are five postulates in total, which are as follows: Postulate 1: A straight line segment can be drawn between any two points.

Postulate 2: Any straight line segment can be extended indefinitely in a straight line.

Postulate 3: Given any straight line segment, a circle can be drawn having the segment as radius and one endpoint as center.

Postulate 4: All right angles are equal to each other.

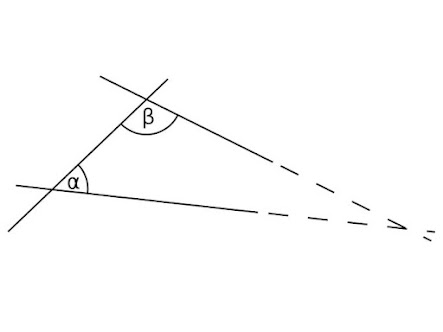
Postulate 5: If a straight line crossing two straight lines makes the interior angles on the same side less than two right angles, then the two straight lines, if extended indefinitely, will meet on that side where the angles are less than two right angles.